Game Theory and Armageddon Bidding

Many rapid chess tournaments these days are run as a series of mini-matches with "armageddon" tiebreakers. In armageddon, players bid for the amount of time they will have for the game, which is usually played without an increment.\footnote{Originally, armageddon games had no increment. But in the current (late 2023) CCT Final they use an increment from move 60 on.} The bidding winner is the player who bids the lower time; they then get to choose which color they will have in the game, with black having draw odds.

This bidding phase adds an interesting new twist to chess competitions. As a game theorist, I decided to dive in, formulate armageddon bidding as a game in itself, see what the results would be, and from them generate insights and advice for tournament players.

TL;DR -- Calculate T*, the time at which you're just indifferent between playing with T* as Black, or playing with White and your opponent starts with T*. Then bid T* or a little bit more, depending on the circumstances.

Setup and Assumptions

For a game theory analysis, you need three elements: players, strategies and outcomes. First, then, we will assume that there are two players, A and B.

Their strategies are time bids tA and tB , with an overall time limit T, typically 5, 10 or 15 minutes. (At first I thought that the bids have to be in the range [0,T], but it seems that players can bid more than T if they want. In fact, Magnus Carlsen once bid 15:01 to ensure that he got the white pieces in his game. See the Comments section below.) Whoever bids the lower amount then gets to choose which color C they will use, with ties broken by some mechanism, such as a coin toss.

As for outcomes, the result of any single game is never certain beforehand, but we can say that there is some probability P that a given player is victorious, given the match parameters. The only assumption in the analysis is that a player's chances of winning increase the more time they start with, and decrease the more time their opponent starts with, regardless of which color they play with. For instance, if Player A has 9 minutes vs. 15 for player B, the time difference is -6 from Player A's point of view. If it’s 12 vs. 15, the difference is -3. If Player A has 15 minutes and Player B has 11, it’s +4, and so on. The assumption is that, no matter which color Player A has for the game, their chances of winning increase as this time differential increases.

So a strategy for Player i is a pair (ti,Ci) giving their time bid and color choice. Then Player A wants to choose a strategy to maximize their probability PA of winning, while Player B wants to minimize it.

Analysis

In game theory, we look for the Nash Equilibrium of a game. These are strategies (tA,CA) for Player A and (tB,CB) for Player B such that neither player can improve their chances of winning by changing strategies. I'll give the results of the analysis as a series of observations.

1. Armageddon bidding is a zero-sum game; what's good for one player is necessarily worse for the other.
2. One consequence of this observation is that there is a particular color C* that both players will pick if they win the bidding -- they will either both choose White or both choose Black. As noted above, in practice Black is almost always chosen due to their having draw odds.
3. The key to the solution is determining what we'll call T*. This is the time at which a player is indifferent between having T* minutes for the game with color C* vs. T for their opponent, or having T themselves and letting their opponent play with time T* and color C*.
4. To determine T*, a player should pick a sample bid and ask themselves which color they would rather play with those time odds. For example, in a 10-minute armageddon, say we start by considering a bid of 6 minutes. The question is, do we think we have a greater chance of winning with 6 minutes versus 10 as Black, or 10 minutes versus 6 as White? If we would choose Black, then we should lower our bid; if white, we should raise it. When the player thinks the have the (more or less) exact same chance winning either way, they have arrived at T*.
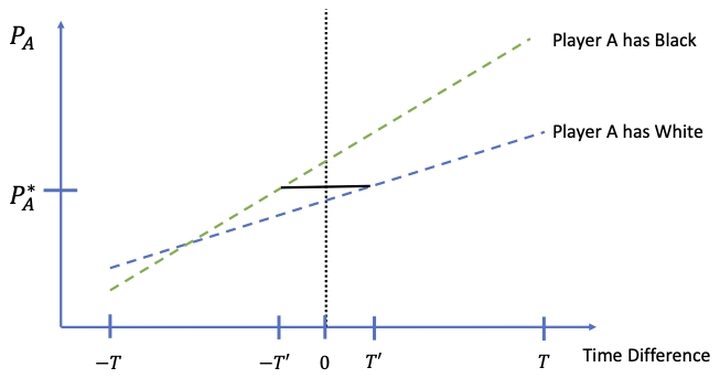
5. It might be a bit surprising that T* is unique and the same for both players, but it follows from the zero-sum nature of the game. The figure below shows the derivation of T* in graphic format. In the graph, T' is the time differential; to get T*, subtract T' from T. So for a 10-minute game with T'=3 minutes, T* would be 7 minutes.
6. The horizontal axis gives the time differential, while the vertical axis is the probability that Player A wins. The blue and green lines show the probability that A wins with a given time differential as White and as Black, respectively. As indicated above, these both rise as the time differential swings more in Player A's favor. Their exact position will depend on the strengths and weaknesses of the two players: which one is more solid with black, which one plays better with little time on their clock, which one has better opening preparation and so can play the first 10-15 moves quickly (if they stay within known theory), and so on. So T* will in general change from game to game.
7. The horizontal line in the middle of the figure is the unique line symmetric about a 0 time differential and equidistant from the two probability curves. This means that the probability PA of Player A winning is the same, whether they win with a bid of T* and take Black, or lose to a bid of T*, play with White and start with T minutes.
8. The Nash Equilibrium of the game, then, is for both players to use the strategy (T*,C*), and the winner is determined by the tie-breaking mechanism. In fact, at the highest levels, the two bids are often quite close to each other, so the players may be arriving at the game theory recommendation intuitively.

(Note: Although game theorists often make predictions that players will use the exact same strategy, this rarely happens in the real world. Hence my surprise and delight that it actually happened in the recent CCT Finals in an armageddon game between Nakamura and MVL. I was very disappointed that the players were forced to bid again, as if the tied outcome was somehow illegitimate and the players should be forced to use sub-optimal strategies. I would have much preferred a coin toss.)

Comments and Suggestions

Now that we know the Nash Equilibrium to the bidding game, what do we do with it? Let's unpack the results a bit.

Practical Bidding Advice

To begin with, once a player figures out what T* is, they should use this as a minimum bid; it makes no sense to bid lower. Assume, for instance, that in a 10-minute game T* is 7:00, and you're thinking of bidding 6:45 instead.

There are three possibilities: a) You would have won with a bid of 7:00 and you still win with 6:45. Then you've just given yourself 15 seconds less for the game. b) You would lose the bidding with both 7:00 and 6:45 because your opponent bid lower than 6:45. Then lowering your bid makes no difference. c) You would have lost with a bid of 7:00 but now win with 6:45 because your opponent bid something like 6:50. Then you are worse off, because, by the definition of T*, your probability of winning with White and 10 minutes vs. 6:50 is higher than the probability of winning with Black with 6:45 vs. 10 minutes. Overall, if lowering your bid below T* makes any difference to the outcomes, it makes you worse off in expectation.

On the other hand, you might consider shading your bid a little higher than T*. Same setup as above, but you're considering a bid of 7:15. Then if you would have won with 7:00 and still win with 7:15, you've given yourself 15 more seconds and come out ahead. However, If you would have won before but lose now, because your opponent bids something like 7:10, then you're worse off. This is an area where psychology and knowing your opponent will help, something more akin to poker than chess. Are they cautious and hesitant to bid too aggressively? Are they inexperienced at armageddon (or haven't read this article!) and not sure how to judge their bids? All these factors might influence your willingness to raise your bid above T*.

Determinants of T*

What factors will affect the magnitude of T*? It's not the relative playing strength of the two players. Look at the figure above and imagine moving both graph lines up and down in tandem. This will move P* higher or lower -- as the lines move up, P* will increase, meaning that Player A is better than Player B and has a higher chance of winning no matter which color they play with. But the length of the middle horizontal line, which is what determines T*, won't necessarily change much.

What would have a significant impact on the length of this middle line is if, say, we leave the lower line fixed and raise the upper line. This corresponds to a higher chance of winning the match if a player has the Black pieces. So the larger the bonus of playing with the preferred color (Black vs. White in most cases), the more aggressively the players should bid.

Remember, though, that there is still only a single, shared value of T*, and players should plan their strategies accordingly. Suppose that one of the two players has a very solid opening repertoire with Black and will be hard to beat (think of Wesley So). Then that player should lower their bids in an attempt to get Black. But their opponent should realize this too and also bid more aggressively, trying to deny the first player the chance to just play solidly with Black and force them to play for a win with White.

Information and Preparation

There's another aspect of the game worth mentioning, which is that so far we have been implicitly assuming full information; that is, all the parameters of the game are equally known to both players. This was never quite correct, and in the modern age of computers and extensive home preparation it's an even more tenuous assumption. (There's a lot to be said here. I'm planning a longer article on Game Theory and Chess that will delve into these issues more thoroughly.)

Say that one of the players, unbeknownst to their opponent, has an opening innovation with White which they think will give them good chances to win the game. Then they might follow the example of Magnus Carlsen who once bid 15:01 for a 15-minute armageddon game just to get the White pieces. Of course, seeing such a suspicious bid, his opponent could try to foil Magnus by choosing the White pieces themselves. But perhaps this is what Magnus was hoping for and was just bluffing with his bid.... Game theory is fun!

Conclusion

This article was written to get straight the theory of armageddon bidding, which is surprisingly subtle and interesting from a game theoretic point of view. Later articles will examine data on players' actual armageddon bids, and more generally the role of game theory in chess. Cheers!

PS -- Here's another version of this post, a bit longer and with more mathematical substance.